Week 2: Math and Art

Math and Art

Anybody who has read a history, art, or math book, has likely come across the ideas of linear perspectives, Da Vinci's Mona Lisa, and fractals, each of which encapsulate the creation of art and science through mathematics. 

I've often walked through art museums and wondered how artists make their work look so real, and one of the most essential methods used is linear perspective demonstrated by Italian Artist Brunelleschi. He demonstrated the intrinsic nature of perspectives by using mirrors to accurately draw the Florence baptistry. His findings were used to develop optics and even telescopes. The importance of his observation is preserved by the essential incorporation of perspective into art and math classes today. 

Linear Perspective: https://encrypted-tbn0.gstatic.com/images?q=tbn:ANd9GcQMKLCjZwjn1PnfdgkPZOVMpgta7bZwezi5doKaSOU2_gK9VC_OzSyhEP5DDCnlwRkNV0g&usqp=CAU

Edwin A. Abbott's Flatland: A Romance of Many Dimensions tells a story of the two-dimensional universe from the perspective of a square who is later introduced to the three-dimensional world by a sphere. Through his stories, Abbott discusses rigid societal perspectives but also exemplifies the intrinsic geometry in art and science, such as through the example of a coin placed on the edge of a table, which becomes an oval and then a line as you lower your gaze at get closer to the coin. This helps visualize through narrative, the importance of expanding perspectives. 

Another widely used mathematical concept is that of the golden ratio, which has been used in multiple in artistic masterpieces including in the creation of the Mona Lisa. In fact, as Vesna suggests, the concept dates back centuries and was used in the construction of the Pyramids of Giza, amongst other important monuments.

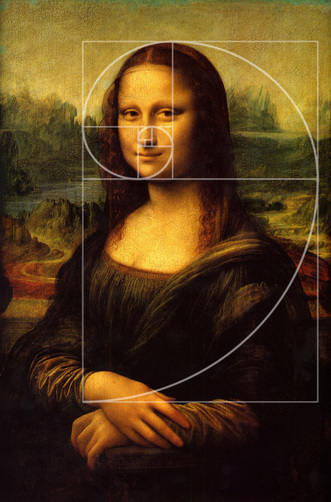Mona Lisa and the Golden Ratio: https://thefibonaccisequence.weebly.com/uploads/1/8/2/8/18283035/9590452.jpg?332

My personal favorite evidence of the intrinsic presence of math and geometry in art and science is the presence of fractals in nature and in art. 

Fractals in Nature: https://media.wired.com/photos/5b337101ce9419115f46ba25/master/w_1600%2Cc_limit/fractal_10.jpg

Mandelbrot describes fractals as an effective example of "painting by numbers". They are observed in nature as a direct manifestation of geometry, and proves wrong anybody who believes math cannot be beautiful and ubiquitous. 

Mathematics is, thus, as relevant to the construction of a painting as it is to the construction of a physics equation. To be able to notice this is to realize the intrinsic connections between the worlds of the arts and the sciences through the fundamentals of mathematics.



Works Cited

Abbott, Edwin A. Flatland: A Romance of Many Dimensions. 1884.

Edgerton, Samuel Y. “Brunelleschi's Mirror, Alberti's Window, and Galileo's 'Perspective Tube'.” História, Ciências, Saúde-Manguinhos, vol. 13, no. suppl, 2006, pp. 151–179., https://doi.org/10.1590/s0104-59702006000500010.

Mandelbrot, Benoit B. “Fractals and an Art for the Sake of Science.” Leonardo. Supplemental Issue, vol. 2, 1989, p. 21., https://doi.org/10.2307/1557938. 

Mauney, Anna Claire. “The Golden Ratio Revealed in 7 Masterpieces.” Art & Object, 6 July 2021, https://www.artandobject.com/slideshows/golden-ratio-revealed-7-masterpieces.

Vesna, Victoria. “Mathematics-PT1-ZeroPerspectiveGoldenMean.mov.” YouTube, YouTube, 9 Apr. 2012, https://www.youtube.com/watch?v=mMmq5B1LKDg.

Comments

Post a Comment

Popular posts from this blog

Week 8: Nanotech + Art

Image
Nanotech + Art Nanotechnology studies and manipulates materials in the nanoscale. It was discussed by Richard Feynman in "There's Plenty of Room at the Bottom" in which he highlighted the "enormous potential" for development in the field of nanosciences (Feynman 1). Since then, we have seen this potential be explored in many disciplines including developments in semiconductors, dyes and pigments, and nanomedicine. Many of these advances have depended on novel approaches to design and manipulate nanostructures, making the involvement of art in the field indispensable.  It is hard to believe what you can't see. The nanoscale is impossible to see, but can be imaged using Scanning Tunneling Microscopy (STM) created by Binnig and Rohrer in 1981 (Chen 573). STM uses a sharp probe close to the surface of the specimen being imaged, and uses electron interactions between the tip and the specimen surface to scan it. This produces a grayscale image which is converted t...
Read more

Week 3: Robotics + Art

Image
Robotics and Art The development of machines from the development of the printing press, has been a topic of marvel but also an issue of debate across disciplines. It played a large role in industrialization and the automation of many labor-intensive tasks in factories. Today, improvements in technology includes artificial intelligence that can produce original material and robots that look human, and the progress is yet to down. However, the initial hesitancy against robotics persists. The Industrial Revolution One of the most important early arguments made against automation was in 1936 by Walter Benjamin. He argued that the authenticity or "aura" of art is depleted by mechanical reproduction. Many people believe creativity in children is hindered by tablets, TVs, and computers. However, by 1995 Douglas Davis contradicted the loss of authenticity that Benjamin described. Davis suggests, instead that the "aura" had been stretched to allow transmission of informatio...
Read more

Event 3: LASER: Decolonizing AI

Image
LASER: De-colonizing AI On Thursday, June 1st, I attended a LASER Talks event on "Decolonizing AI". The Leonardo/ISAST LASER Talks program brings together people from various disciplines including art and science to give informal presentations about their work and "encourage contribution to the cultural environment of a region". The talk focused on the western-centric development of AI and the potential for machines to inherit the same imperialism and colonialism present in many fields, even today. Amir Baradaran is an augmented reality (AR)tist that discussed the impact of AI on the ecosystem, and more specifically, the art ecosystem. He cautioned about AI becoming the art or the artist but also encouraged the use of AI to help create and innovate.  The talk I was most intrigued by, however, was by the Armenian writer and artist Mashinka Firunts Hakopian, who discussed the inheritance of historically discriminatory and euro-centric views by artificial intelligence....
Read more